Ex 5.3 Class 10 | Ex 5.3 class 10 maths solutions
Ex 5.3 Class 10
1. Find the sum of the following APs:
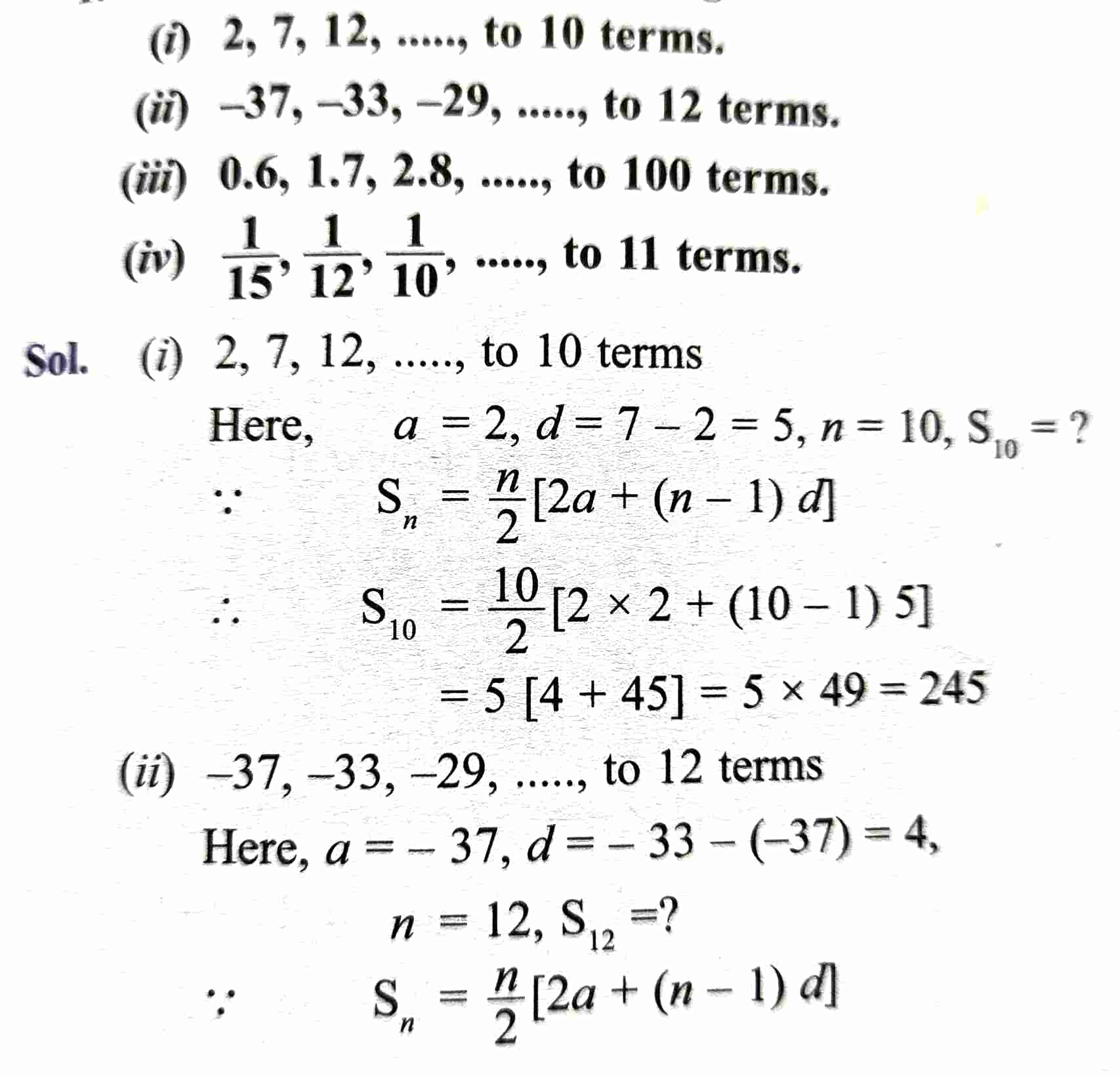
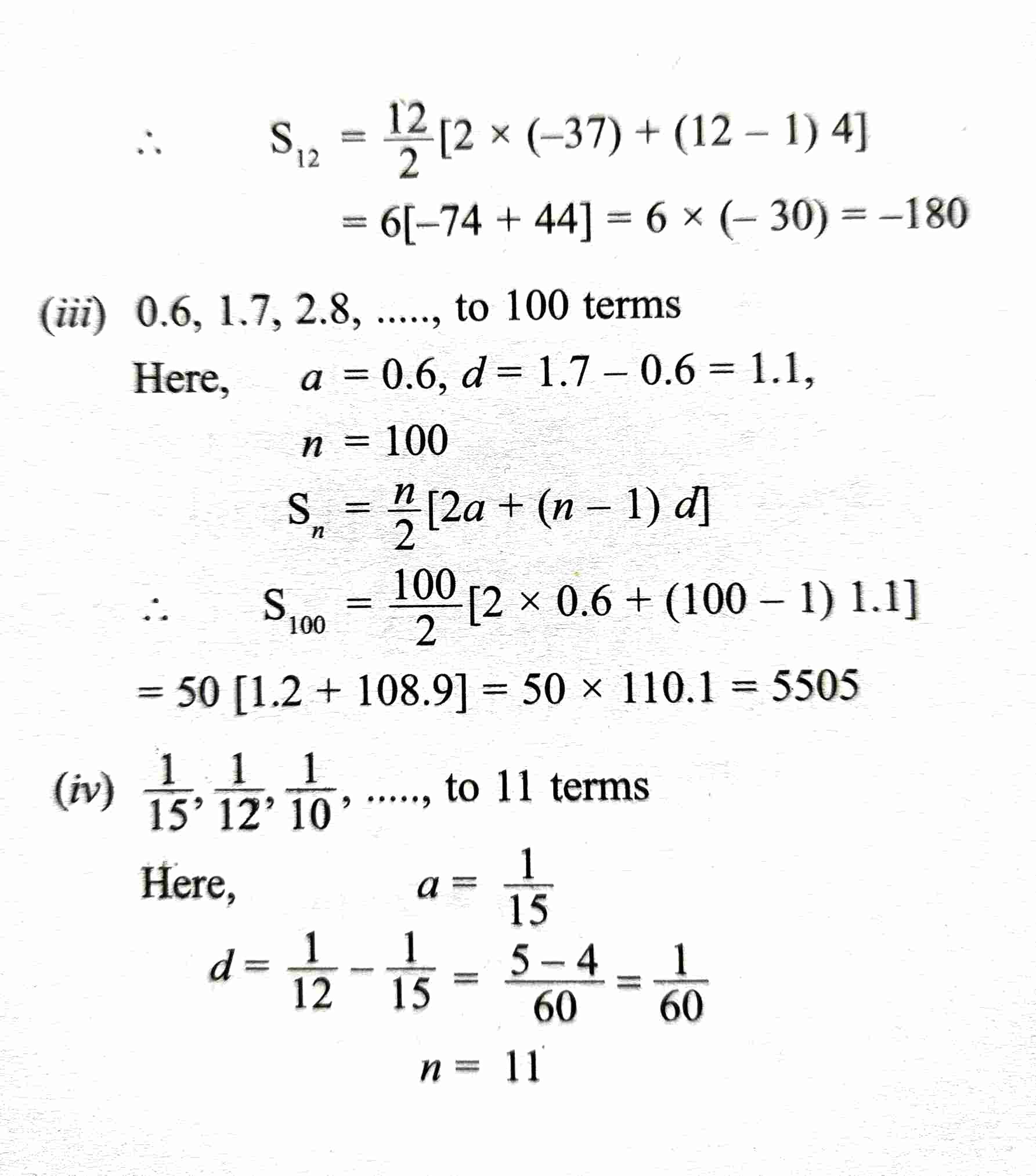
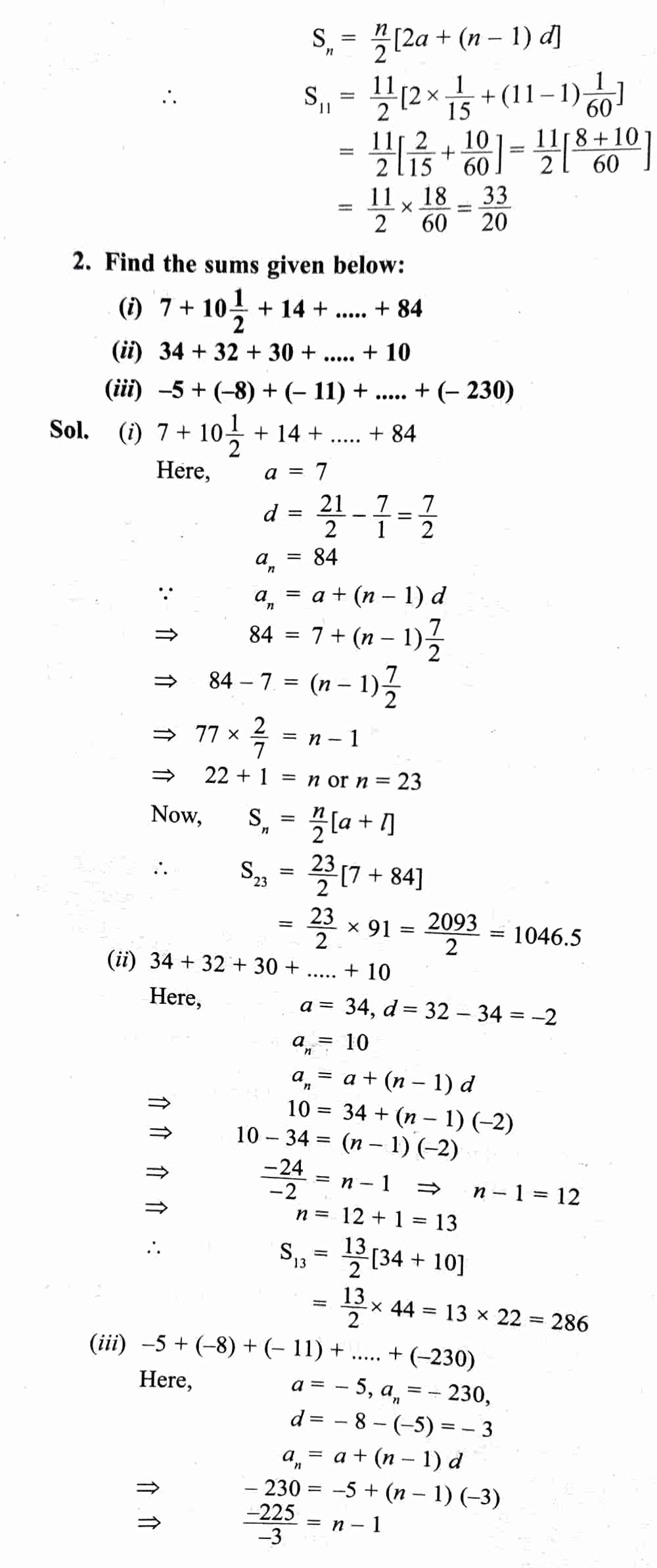
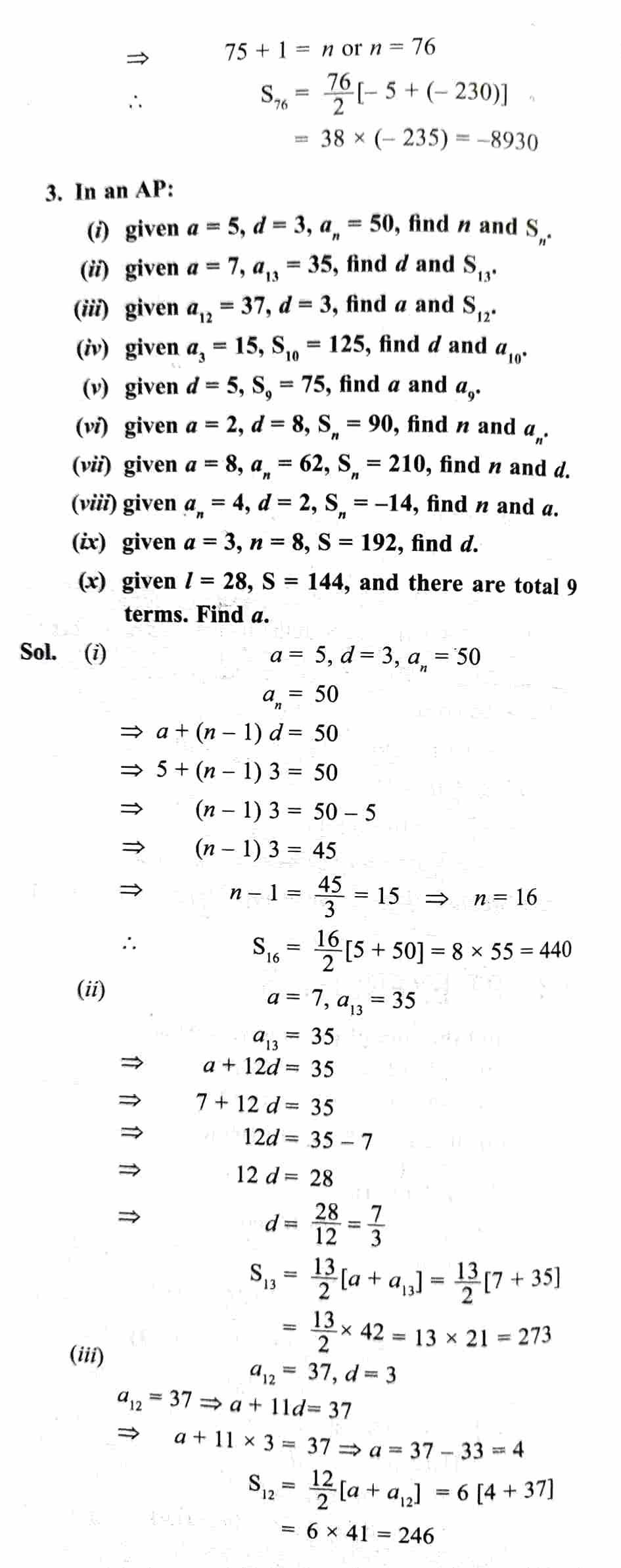
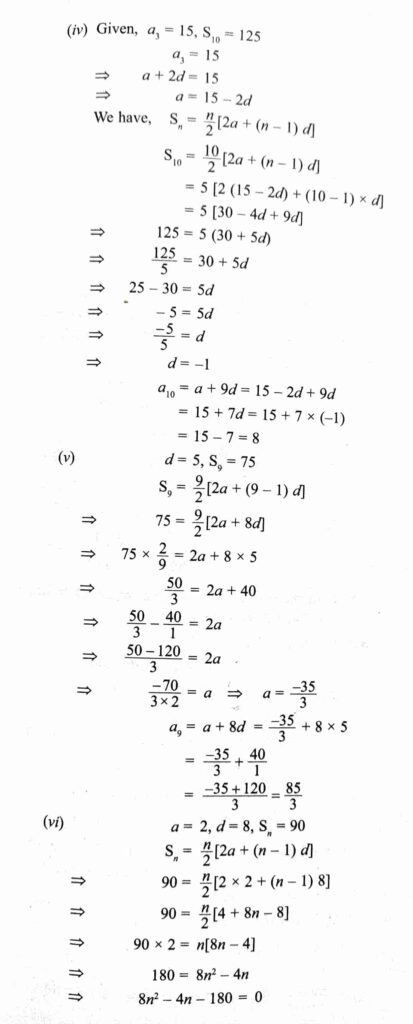
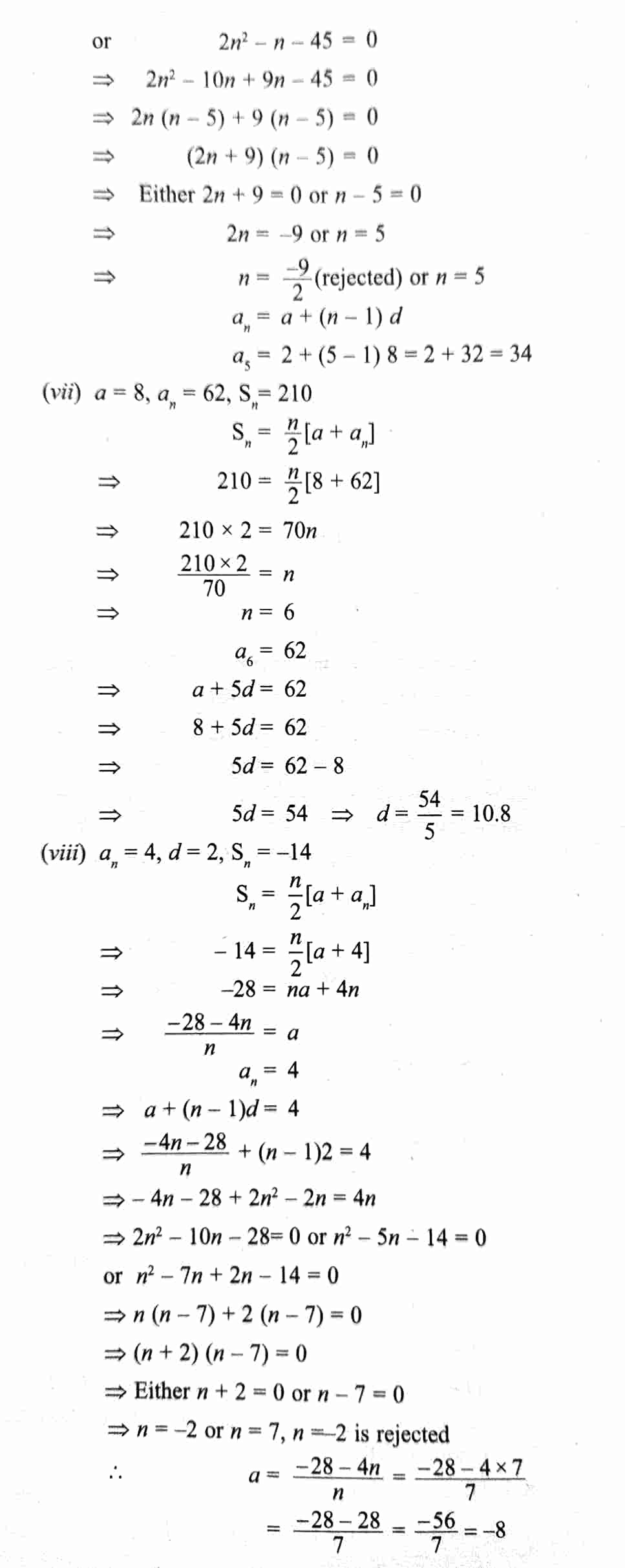
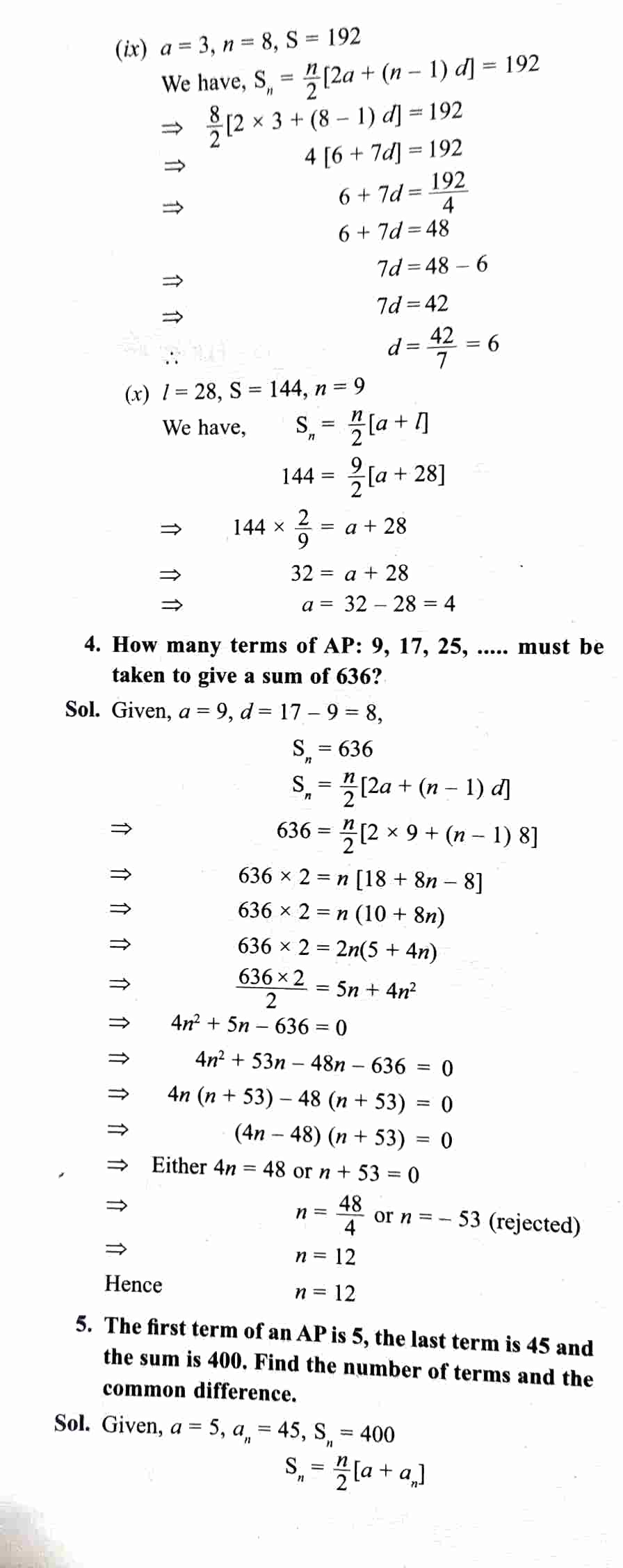

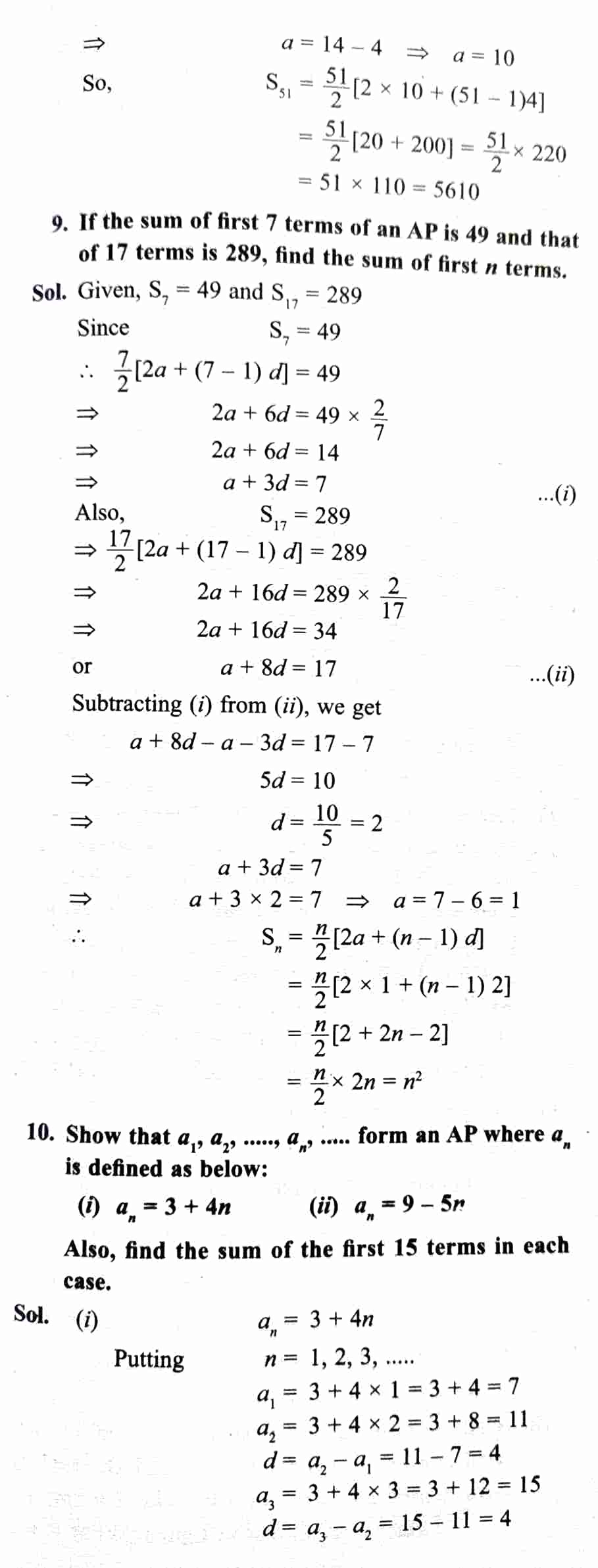



1. Find the sum of the following APs:
2. Find the sums of given below
3. In an AP:
4. How many terms of AP: 9, 17, 25,… Must be taken to give a sum of 636
5. The first term of an ap is 5 the last term is 45 and the sum is 400 find the number of terms and the common difference
6. The first and the last terms of an ap are 17 and 350 respectively if the common difference is 9 how many terms are there and what is their sum
7. Find the sum of first 22 terms of an ap in which D = 7 and 22nd term is 149
8. Find the sum of first 51 terms of an ap whose second and third terms are 14 and 18 respectively
9. If the sum of first 7 terms of an ap is 49 and that of 17 terms is 289 find the sum of first n terms
10. Show that a1, a2,…., an,…… Form an ap where an is defined as below
11. If the sum of the first n terms of an AP is 4n-4n2, what is the first term (that is S1)? What is the sum of first two terms what is the second term similarly find the third and the 10th and the nth term
12. Find the sum of the first 40 positive integers divisible by 6
13. Find the sum of the first 15 multiples of 8
14. Find the sum of the odd numbers between 0 and 50
15 a contract on construction job specifies a penalty for delay of completion beyond a certain date as follows rupees 200 for the first day rupees 250 for the second day rupees for the third day extra the penalty for each succeeding day being rupees 50 more than for the proceeding day how many much money the contractor has to paynalty if he has delayed the work for by 30 days
16. A sum of rupees 700 is to be used to give 7 cash prices to students of a school for their overall academic performance if each price is rupees 20 less than its preceding price find the value of each of the prices
17. In a school students thought of planting trees in and around the school to reduce air pollution it was decided that the number of trees that each section of each class will plant will be the same as the class in which they are studying a section of class 1 will plant one tree a section of class 2 will plant two trees and so on till class 12 there are three sections of each class how many trees will be planted by the students
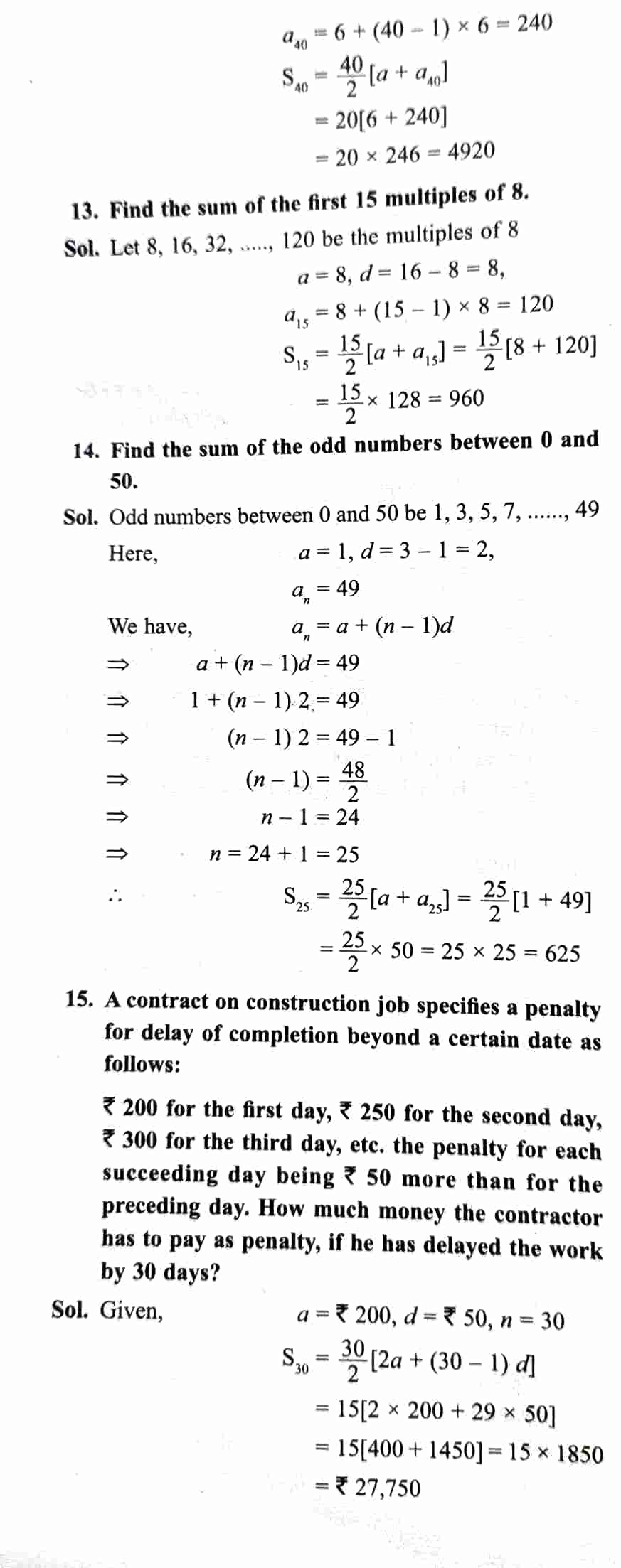
18. A spiral is made up of successive semi circles with centres alternately at A and B starting with the centre at a of radii 0.5 CM, 1.0 CM 1.5 CM 2.0,…. As shown in figure 5.4 what is the total length of such spiral made up of 13 constructive semi circles
19. 200 logs are stacked in the following manner 20 logs in the bottom rope 19 in the restro and 18 in the next row to it and so on in how many rows are the 200 logs placed and how many logs are in the top now
20. In a potato race a bucket is placed at the starting point which is 5 m from the first potato and the other potatoes are placed 3 m apart in a straight line there are 10 potatoes in the line.
Arithmetic Progression is a mathematical concept that deals with a sequence of numbers in which each term is the sum of the previous term and a constant value, called the common difference. Arithmetic Progression is widely used in various fields of mathematics, such as algebra, geometry, and calculus. Additionally, it is used in practical applications such as financial calculations, where the interest rate remains constant, and in physics, where an object’s velocity is either increasing or decreasing at a constant rate.
To understand the concept of Arithmetic Progression, consider an example of the following sequence of numbers: 1, 4, 7, 10, 13, 16, 19, 22, 25, 28, and 31. This sequence is an example of Arithmetic Progression, where the common difference is 3, and the first term is 1.
The formula to find the nth term of an Arithmetic Progression is a + (n-1)d, where a is the first term, n is the number of terms, and d is the common difference. For instance, to find the 10th term of the above sequence, we use the formula: 1 + (10-1)3 = 28.
We can also find the sum of n terms in an Arithmetic Progression by using the formula Sn = n/2[2a + (n-1)d]. For example, to find the sum of the first 10 terms of the above sequence, we use the formula: S10 = 10/2[2(1) + (10-1)3] = 155.
Arithmetic Progression has several properties that aid in solving problems related to it. One of the critical properties is that the sum of the first n terms of an Arithmetic Progression is equal to the product of the average of the first and last terms and the number of terms, which is given by the formula Sn = n/2[a + l], where l is the last term.
Another useful property of Arithmetic Progression is that if the sum of the first n terms is S, and the sum of the first m terms is T, where m<n, then the sum of the remaining (n-m) terms is (S-T). This property is helpful in problems that require finding the sum of the remaining terms of an Arithmetic Progression.
Arithmetic Progression also has a concept of the nth term from the end, which is calculated using the formula: l – (n-1)d, where l is the last term. For instance, to find the 5th term from the end of the above sequence, we use the formula: 31 – (5-1)3 = 19.
In conclusion, Arithmetic Progression is a fundamental concept in mathematics that has various applications in different fields. It is vital to understand the formulae and properties of Arithmetic Progression to solve problems related to it effectively.
Ncert Solutions Class 10 Maths Exercise 1.1 https://10thmathsguide.com/exercise-11-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 1.2
Ncert Solutions Class 10 Maths Exercise 2.1 https://10thmathsguide.com/exercise-21-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 2.2
Ncert Solutions Class 10 Maths Exercise 3.1 https://10thmathsguide.com/exercise-31-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 3.2 https://10thmathsguide.com/exercise-32-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 3.3 https://10thmathsguide.com/exercise-33-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 4.1 https://10thmathsguide.com/exercise-41-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 4.2 https://10thmathsguide.com/exercise-42-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 4.3 https://10thmathsguide.com/exercise-43-class-10-maths/
MCQ VIDEOS ALL CHAPTERS CLASS 10 MATHShttps://www.youtube.com/watch?v=-eBlHyBxjLg&list=PL2uPMjJCHErQZZNipbsnagBqPrCU_WRN8
Class 10 ex 5.3,
Ex 5.2 class 10,
Ex 5.3 ,
5.2 maths class 10,
class 10 maths ex 5.3 ,
class 10 maths ch 5 ex 5.3 ,
ex 5.3 class 10 maths solutions ,
Exercise 5.2 class 10 maths,
Ex 5.3 class 10 maths solutions ,
ex 5.3 class 10,
maths ex 5.3 class 10 ,
class 10 ch 5 ex 5.3




