Ex 5.1 class 10 | Ex 5.1 class 10 maths solutions
Ex 5.1 class 10
1. In which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(i) The taxi fare after each km when the fare is rupees 15 for the first km and Rs 8 for each additional km.
(ii) The amount of air present in a cylinder when a vacuum pump removes 1/4 th of the air remaining in the cylinder at a time.
(iii) The cost of digging a well after every meter of digging, when it cost rupees 150 for the first metre and rises by rupees 50 for each subsequent metre.
(iv) The amount of money in the account every year, when rupees 10000 is deposited at compound interest at 8% per annum.

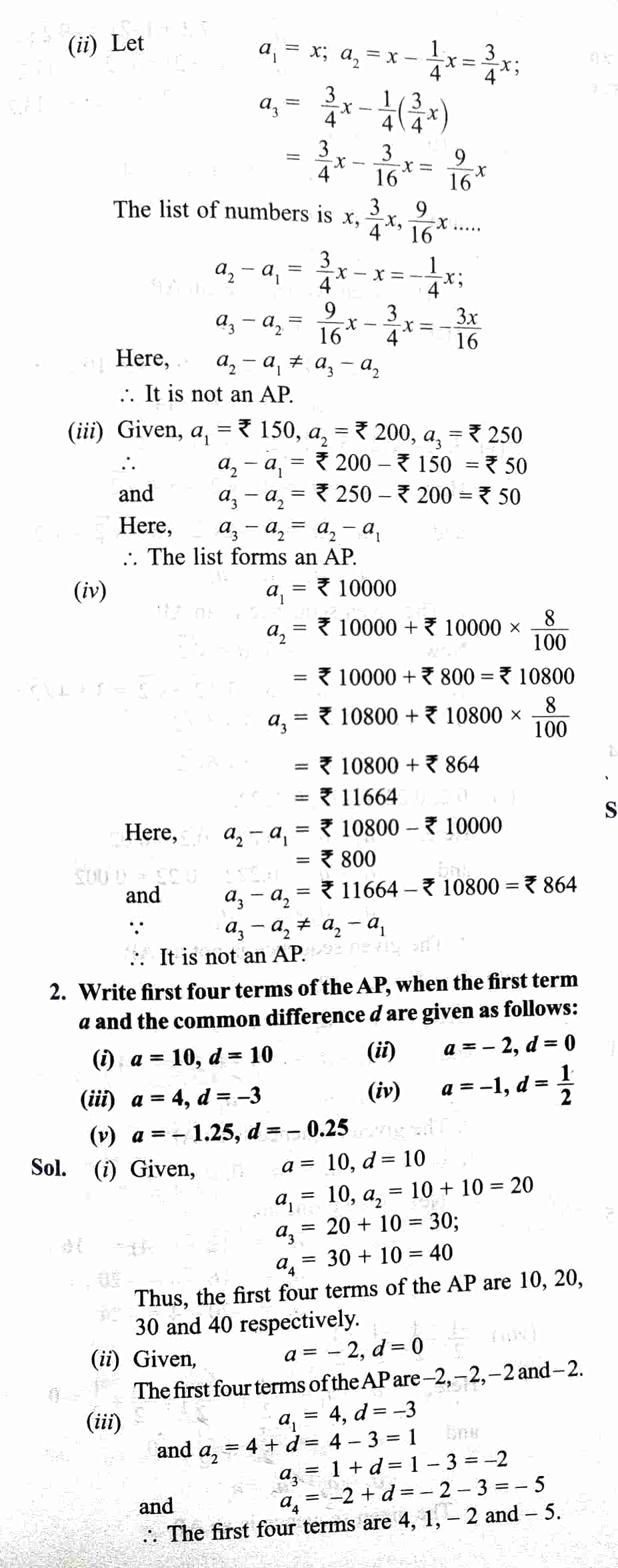


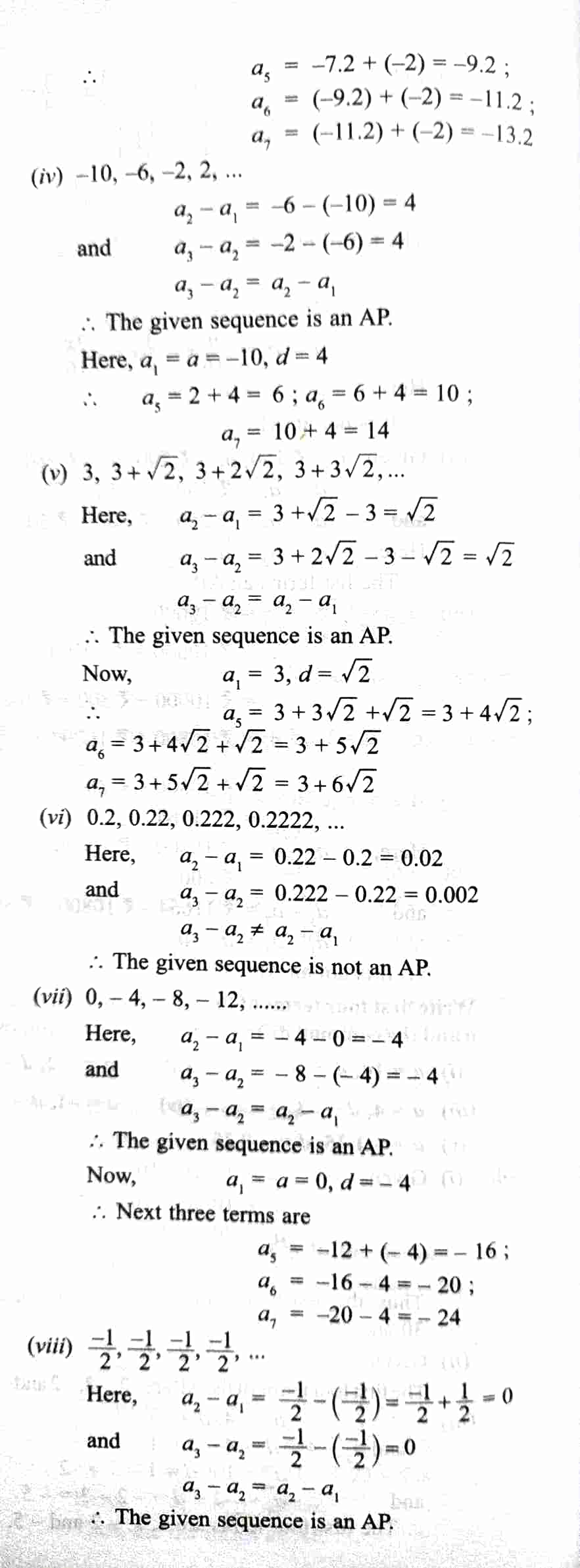


Arithmetic Progression (AP) is a fundamental mathematical concept that finds widespread application across fields such as finance, economics, physics, and computer science. In AP, the difference between any two consecutive terms in a sequence is constant, and this constant difference is referred to as the common difference, denoted by d.
The arithmetic progression is typically represented as {a, a+d, a+2d, a+3d,….}, where a is the first term and d is the common difference. The nth term of an AP is given by the formula tn = a + (n-1)d, where n represents the number of terms in the sequence.
APs find extensive use in everyday life, such as calculating the money spent or saved over a period, the distance covered by an object in a given time, or temperature variation over time.
The most significant feature of APs is their simple summation process. The sum of the first n terms of an AP can be calculated by using the formula Sn = n/2(2a + (n-1)d). This formula can be applied to any number of terms in the sequence. For example, if one wants to find the sum of the first ten terms of an AP with the first term as 3 and the common difference as 2, then the formula Sn = 10/2(2(3) + (10-1)2) can be used, which results in 55.
Another vital property of APs is their ability to determine the missing terms in a sequence. The formula tn = a + (n-1)d can be utilized to calculate the missing terms if we know some terms of an AP and the common difference. For instance, if the first term is 2, the common difference is 3, and we want to determine the 7th term, the formula t7 = 2 + (7-1)3 can be used, which yields 20.
Class 10 Mathematics NCERT textbook covers Arithmetic Progressions in . In Ex 5.1 class 10. The chapter introduces APs and the formulas for finding the nth term and sum of the first n terms. Additionally, Exercise 5.1 class 10 maths comprises examples and exercises to enable students to grasp the concept and apply it to real-life problems.
The chapter discusses the sum of the first n odd natural numbers and the sum of the first n even natural numbers, which are special cases of APs, where the first term is either 1 or 2, and the common difference is 2. The formulas to determine the sum of these special APs are also provided in the chapter.
Furthermore, the chapter explains the sum of the squares and cubes of the first n natural numbers, which are examples of APs with common differences of 2 and 3, respectively. The formulas to determine the sum of these APs are also provided in the chapter.
Finally, the chapter provides several exercises to help students practice the concepts covered in the chapter. These exercises involve determining the missing terms of an AP, finding the sum of the first n terms of an AP, and determining the sum of the first n odd or even natural numbers.
In conclusion, Ex 5.1 of Class 10 Mathematics textbook is a crucial chapter that introduces students to Arithmetic Progression and its varied applications in real-life scenarios. The chapter provides students with the tools to solve practical problems involving APs and helps them develop their mathematical skills.
Class 10 ex 5.1,
Ex 5.1 class 10,
Ex 5.1,
5.1 maths class 10,
class 10 maths ex 5.1 ,
class 10 maths ch 5 ex 5.1 ,
ex 5.1class 10 maths solutions ,
Exercise 5.1 class 10 maths,
Ex 5.1 class 10 maths solutions ,
ex 5.1 class 10,
maths ex 5.3 class 10 , class 10 ch 5 ex 5.3
Ncert Solutions Class 10 Maths Exercise 1.1 https://10thmathsguide.com/exercise-11-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 1.2
Ncert Solutions Class 10 Maths Exercise 2.1 https://10thmathsguide.com/exercise-21-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 2.2
Ncert Solutions Class 10 Maths Exercise 3.1 https://10thmathsguide.com/exercise-31-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 3.2 https://10thmathsguide.com/exercise-32-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 3.3 https://10thmathsguide.com/exercise-33-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 4.1 https://10thmathsguide.com/exercise-41-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 4.2 https://10thmathsguide.com/exercise-42-class-10-maths/
Ncert Solutions Class 10 Maths Exercise 4.3 https://10thmathsguide.com/exercise-43-class-10-maths/
MCQ VIDEOS ALL CHAPTERS CLASS 10 MATHShttps://www.youtube.com/watch?v=-eBlHyBxjLg&list=PL2uPMjJCHErQZZNipbsnagBqPrCU_WRN8
